iopcountry.blogg.se

(c) Flywheel mass and radius should both be much greater, allowing for a lower spin rate (angular velocity). The radial acceleration at the edge of the disk is > 50,000 gs. Because the force is perpendicular to r, an acceleration \boldsymbol$$ (b) This angular velocity is very high for a disk of this size and mass. To develop the precise relationship among force, mass, radius, and angular acceleration, consider what happens if we exert a force F on a point mass m that is at a distance r from a pivot point, as shown in Figure 2. If you push on a spoke closer to the axle, the angular acceleration will be smaller. The more massive the wheel, the smaller the angular acceleration. The greater the force, the greater the angular acceleration produced. Force is required to spin the bike wheel. There are, in fact, precise rotational analogs to both force and mass. That equation did not explicitly include the amount of frictional energy loss. These relationships should seem very similar to the familiar relationships among force, mass, and acceleration embodied in Newton’s second law of motion. (7) Equation (8) was used to calculate moments of inertia. The first example implies that the farther the force is applied from the pivot, the greater the angular acceleration another implication is that angular acceleration is inversely proportional to mass. Furthermore, we know that the more massive the door, the more slowly it opens. For example, we know that a door opens slowly if we push too close to its hinges. In fact, your intuition is reliable in predicting many of the factors that are involved. If you have ever spun a bike wheel or pushed a merry-go-round, you know that force is needed to change angular velocity as seen in Figure 1.

Study the analogy between force and torque, mass and moment of inertia, and linear acceleration and angular acceleration.

O is the centre of the circular section as displayed in following figure. Let us consider one hollow circular section, where we can see that D is the diameter of main section and d is the diameter of cut-out section as displayed in following figure.

Understand the relationship between force, mass and acceleration. Today we will see here the method to determine the moment of inertia of a hollow circular section with the help of this post.

It may be noted that moment of inertia depends not only on mass m but also on r². You have already learned about inertia in linear systems an object in motion will continue in motion as long as there is no net force acting on it. For a planar mass density rho (r, phi), I integrate (rho (r, phi)r2 d (area)) where r j distance from mass m j to the center of mass. The moment of inertia plays the same role in angular motion as the mass in linear motion. The definition of moment of inertia ‘I’ is: For ’n’ discrete masses, I sum (m j r j2, j 1, n) where r j distance from mass m j to the center of mass. The quantity mr² is known as the moment of inertia and is represented by I. One radian is that angle subtended at the center of a circle by an arc equal in length to. Here F is replaced by τ, a by α and m by mr². Which is the rotational analog of Newton’s second law of motion? This can be done by multiplying both sides of the above equation by r.

This righting force has the effect of causing the rotational axis to execute a circular, wobbling motion.

In doing so the force will cause the mass to rotate about O.Since tangential acceleration is related to angularĪs the turning effect is produced by torque τ, it would, therefore, be better to write the equation for rotation in terms of torque. This would be a least energy configuration. A force F is acting on the mass perpendicular to the rod and hence this will accelerate the mass according to: Let us assume that the bearing at the pivot point O is frictionless. Moment of inertia equationĬonsider a mass m attached to the end of a massless rod. Hence the kinetic energy of a body rotating about a fixed axis with angular velocity ω is ½ω², which corresponds to ½mv² for the kinetic energy of a body of mass m translated with velocity v.See also Routh’s rule the theorem of parallel axes.

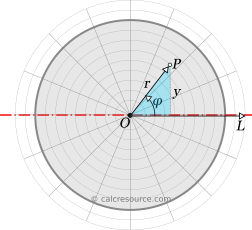
The moment of inertia plays a role analogous to that of the inertial mass in the case of rectilinear and uniform motion. It is the scalar value of the longitudinal angular momentum of a rigid solid.įor a rigid body moving about a fixed axis, the laws of motion have the same form as those of rectilinear motion, with the moment of inertia replacing mass, angular replacing linear velocity, angular momentum replacing linear momentum, etc.

The moment of inertia reflects the mass distribution of a body or a system of rotating particles, with respect to an axis of rotation. The moment of inertia only depends on the geometry of the body and the position of the axis of rotation, but it does not depend on the forces involved in the movement.